- Главная
- Разное
- Образование
- Спорт
- Естествознание
- Природоведение
- Религиоведение
- Французский язык
- Черчение
- Английский язык
- Астрономия
- Алгебра
- Биология
- География
- Геометрия
- Детские презентации
- Информатика
- История
- Литература
- Математика
- Музыка
- МХК
- Немецкий язык
- ОБЖ
- Обществознание
- Окружающий мир
- Педагогика
- Русский язык
- Технология
- Физика
- Философия
- Химия
- Шаблоны, фоны, картинки для презентаций
- Экология
- Экономика
Презентация, доклад по геометрии на тему Parallelepiped and block
Содержание
- 1. Презентация по геометрии на тему Parallelepiped and block
- 2. There is a certain analogy between the
- 3. Parallelepipeds are frequently encountered among crystals. They
- 4. If you place through this point planes
- 5. A straight parallelepiped with rectangular base planes
- 6. Planes of symmetry pass parallel to the
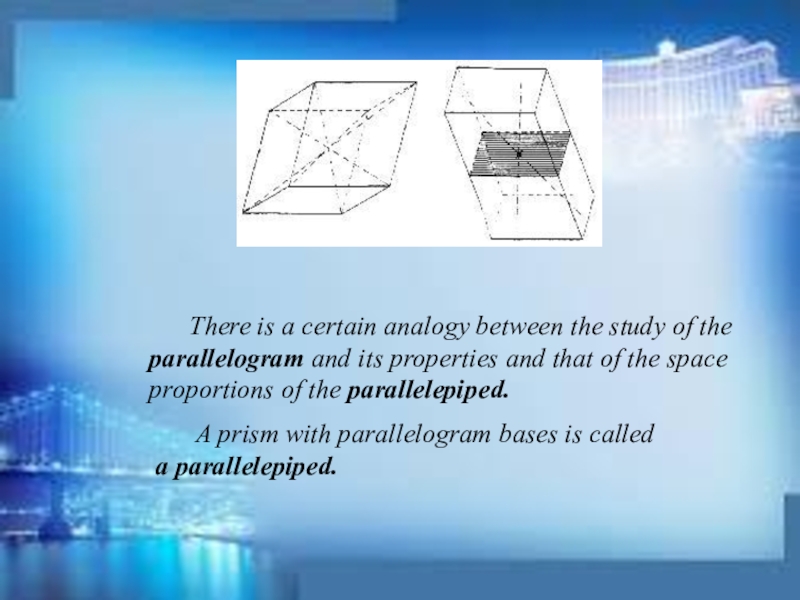
There is a certain analogy between the study of the parallelogram and its properties and that of the space proportions of the parallelepiped. A prism with parallelogram bases is called a parallelepiped.
Слайд 2 There is a certain analogy between the study of the parallelogram
and its properties and that of the space proportions of the parallelepiped.
A prism with parallelogram bases is called
a parallelepiped.
Слайд 3 Parallelepipeds are frequently encountered among crystals. They can be generated by
parallel displacement of a parallelogram in a direction, which does not coincide with its planes. Its six side faces are parallelograms, which are in pairs parallel and congruent. Each of these pairs can serve as base plane. There are three groups of four parallel, congruent edges. Three unequal edges radiate from each corner.
A parallelepiped has four body diagonals which join opposite corners. In each diagonal cut of the parallelepiped, formed by two opposite sides and two side diagonals, lie two of these diagonals. However, since every diagonal belongs simultaneously to three of the existing six diagonal cuts, you have the Rule:
The body diagonals intersect in a point and bisect each other.
Слайд 4 If you place through this point planes parallel to the base
planes, you create congruent, parallel layers, whence all cross lines are bisected by these layers. Hence also all cross lines, which pass through the intersection of the diagonals, are bisected there, and you have the Rule:
The intersection of the diagonals is the centre of a parallelepiped, which is centrally symmetric.
Слайд 5 A straight parallelepiped with rectangular base planes is a block. At the
corners of a block, the angles between the edges are right and so are those of the planes. Diagonal cuts yield rectangles, because the edges AD and BC are perpendicular to the front and back planes, and therefore are also perpendicular to AB and CD; the angles in ABCD are right, because it is a rectangle. The body diagonals AC and BD are equal, as diagonals in a rectangle. Since, as in a parallelepiped, every diagonal belongs to three diagonal cuts, one has the Rule:
The body diagonals of a block are equal; you can describe a sphere around a block.
Слайд 6 Planes of symmetry pass parallel to the sides through the centre
of a block. They are perpendicular to each other. You can generate a block by reflection of a point in three mutually perpendicular planes.
A block has three, doubly counted body axes, namely the connecting lines of the centres of opposite faces, that is, rotations by 180° and 360° about these axes transform it into itself. The body axes are the intersections of the symmetry planes, whence they pass through the centre of the block.